Last Updated: Monday, February 4, 2013
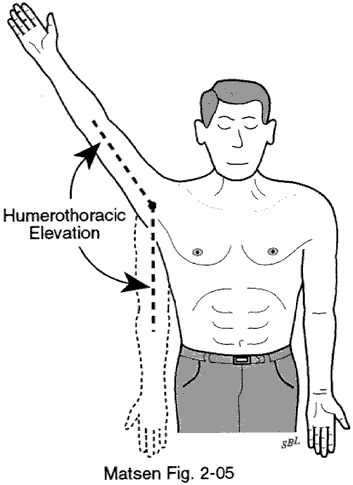
Postion of the arm relative to the chest
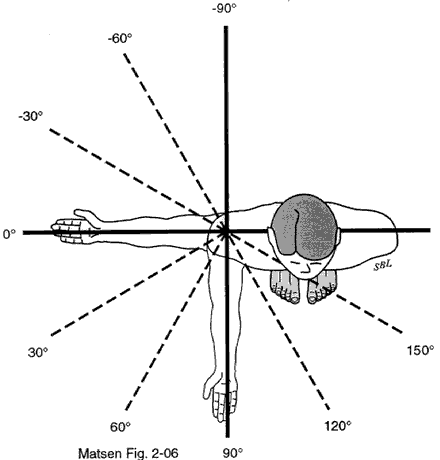
The natural reference lines for describing humerothoracic positions (the position of the arm relative to the chest) are the axis of the humeral shaft and the longitudinal axis of the thorax. The angle between these lines is the angle of humerothoracic elevation. The plane containing these two lines is the plane of humerothoracic elevation. The plane of elevation is identified in relation to a reference plane the coronal plane of the thorax. Using this simple method we can define any position of the humerus in reference to the thorax with only two numbers the angle and the plane of humerothoracic elevation.
The table below lists the average humerothoracic positions for eight common functional positions measured in vivo. The data demonstrate that the humerus functions in a wide range of thoracic planes from minus 88 to plus 124 degrees.
Position Plane of elevation Angle of elevation
| Cross body adduction | 124 | 90 |
| Washing axilla | 104 | 52 |
| Eating | 87 | 52 |
| Maximal elevation | 55 | 148 |
| Combing hair | 54 | 112 |
| Maximum reach up back | -69 | 56 |
| Reaching perineum | -86 | 38 |
| Maximum extension | -88 | 55 |
Angle of humerothoracic elevation
Coronal plane of the thorax
Displaying humerothoracic positions
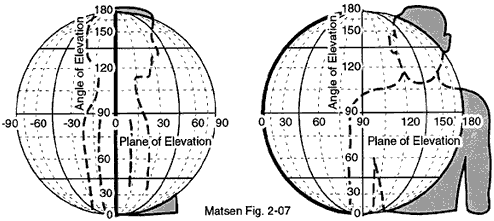
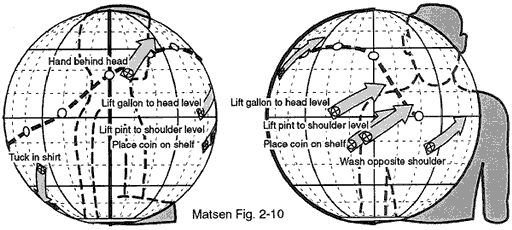
The global diagram is an effective method for displaying humerothoracic positions because it allows presentation of both the planes of elevation ("longitudes") and the angles of elevation ("latitudes"). The "South Pole" of the globe represents zero degrees of elevation. The range of possible positions and the positions required for various activities can be demonstrated on global diagrams. Note that the maximal elevation in the different planes defines the envelope of humerothoracic motion available to this shoulder. The positions used for the Simple Shoulder Test functions lie within this envelope.
By using an arrow pointing in the direction of the anterior humerus (the direction of the forearm if the elbow was flexed to 90ΒΌ) the global diagram also provides a method for indicating the rotational orientation of the arm at maximal elevation and for carrying out the functions of the Simple Shoulder Test.
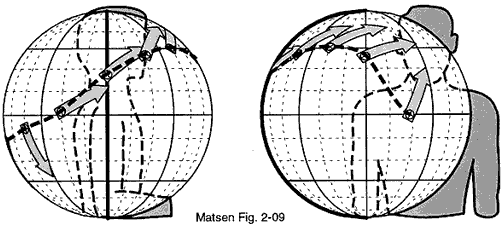
The details of sequential humeral motions for example during a throw can be indicated on a global diagram as a series of points and arrows.
Codman's paradox
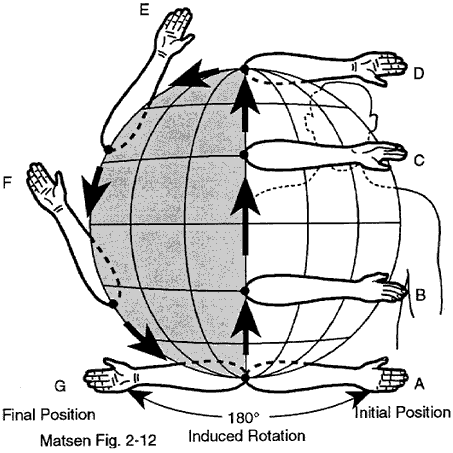
Codman proposed that the completely elevated humerus could be shown to be in either extreme external rotation or in extreme internal rotation by lowering it either in the coronal or sagittal plane respectively without allowing rotation about the humeral shaft axis. We can use the global diagram to examine Codman's paradox:
Carry out the movement sequence described below without allowing rotation about the humeral shaft axis:
- Place the arm at the side with the forearm internally rotated across the stomach.
- Elevate the arm 180 degrees in the plus 90 degree thoracic (sagittal) plane.
- Lower the arm 180 degrees to the side in the 0 degree (coronal) plane.
ote that the forearm now points 180 degrees from its original position. This entire motion can be drawn on a global diagram. The fraction of the surface area of the sphere that is enclosed by this path of motion is 1/4.
This result demonstrates the relationship between enclosed area and rotation. The area of a unit sphere is 4 pi. One-fourth of this is pi; 360 degrees of rotation is equal to 2pi; thus pi is equal to 180 degrees of rotation. Here we see that a humeral path without rotation about the humeral shaft axis circumscribing one-fourth of a sphere results in an induced rotation of 180 degrees.
This relationship between area and induced rotation holds true for any sequence of motions in a closed path in which there is no rotation about the humeral shaft axis. From this relationship we can see that the apparent paradox of induced rotations on Codman's motions is a property of motion on the surface of a sphere and not a paradox at all!
Global diagram
Activities demonstrated
on global diagram
Indicating the rotational orientation
of the arm at maximal elevation
Simple shoulder test
Series of points and arrows
Codman's paradox movement sequence
